難問の積分を解いてみた
問題 \( I=\displaystyle \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{x^2+1+\sqrt{x^4+x^2+1}} \> dx \)を求めよ。
まず,
\[ \begin{array}{r@{\>}l}
x^4+x^2+1 & =x^4+2x^2+1-x^2 \\
& = (x^2+1)^2-x^2 \\
& = \{ (x^2 + 1)+x \}\{ (x^2 + 1)-x \} \\
& = (x^2+x+1)(x^2-x+1)
\end{array} \]
と変形できるので,
\[ \displaystyle I=\int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{x^2+1+\sqrt{(x^2+x+1)(x^2-x+1)}} \> dx \]
二重根号を外すことを考えて,
\[ \begin{array}{rl}
I & \displaystyle =\int_{-\frac{1}{2}}^{\frac{1}{2}} \dfrac{\sqrt{2x^2+2+2\sqrt{(x^2+x+1)(x^2-x+1)}}}{\sqrt{2}} \> dx \\
& \displaystyle = \dfrac{1}{\sqrt{2}} \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{(x^2+x+1)+(x^2-x+1)+2\sqrt{(x^2+x+1)(x^2-x+1)}} \> dx \\
& \displaystyle = \dfrac{1}{\sqrt{2}} \int_{-\frac{1}{2}}^{\frac{1}{2}} (\sqrt{x^2+x+1}+\sqrt{x^2-x+1}) \> dx \\
& \displaystyle = \dfrac{1}{\sqrt{2}} \left( \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{x^2+x+1} \> dx + \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{x^2-x+1}) \> dx \right) \\
\end{array}\]
ここで,ルートの中の平方完成を考えて,
\[ \begin{array}{rl}
I & \displaystyle = \dfrac{1}{\sqrt{2}} \left\{ \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{ \left( x + \dfrac{1}{2} \right)^2+\dfrac{3}{4}} \> dx+\int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{ \left( x-\dfrac{1}{2} \right)^2+\dfrac{3}{4}} \> dx \right\} \\
\end{array}\]
ここで,\(x+\dfrac{1}{2}=\dfrac{\sqrt{3}}{2}\tan{\theta_1}\),\(x-\dfrac{1}{2}=\dfrac{\sqrt{3}}{2}\tan{\theta_2}\)とする。
また,\(\tan t = \dfrac{2}{\sqrt{3}} \left(0 \leqq t \leqq \dfrac{\pi}{2}\right)\)を満たす \( t \) を \(\alpha\) とすると,
\[\begin{array}{rl}
\dfrac{dx}{d\theta_1} & = \dfrac{\sqrt{3}}{2}\dfrac{1}{\cos^2\theta_1} \\
\dfrac{dx}{d\theta_2} & = \dfrac{\sqrt{3}}{2}\dfrac{1}{\cos^2\theta_2}
\end{array}\]
\[\begin{array}{c|ccc}
x & -\dfrac{1}{2} & \rightarrow & \dfrac{1}{2} \\ \hline
\theta_1 & 0 & \rightarrow & \alpha
\end{array} \hspace{5mm}
\begin{array}{c|ccc}
x & -\dfrac{1}{2} & \rightarrow & \dfrac{1}{2} \\ \hline
\theta_2 & -\alpha & \rightarrow & 0
\end{array}\]
となるので,
\[ \begin{array}{rl}
I & \displaystyle = \dfrac{1}{\sqrt{2}} \left( \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{ \dfrac{3}{4}\tan^2\theta_1+\dfrac{3}{4}} \> dx+\int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{ \dfrac{3}{4}\tan^2\theta_2+\dfrac{3}{4}} \> dx \right) \\
& \displaystyle = \dfrac{1}{\sqrt{2}} \left( \int_{-\frac{1}{2}}^{\frac{1}{2}} \dfrac{\sqrt{3}}{2}\sqrt{1+\tan^2\theta_1} \> dx+\int_{-\frac{1}{2}}^{\frac{1}{2}} \dfrac{\sqrt{3}}{2}\sqrt{1+\tan^2\theta_2} \> dx \right) \\
& \displaystyle = \dfrac{\sqrt{3}}{2\sqrt{2}} \left( \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{\dfrac{1}{\cos^2\theta_1}} \> dx+\int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{\dfrac{1}{\cos^2\theta_2}} \> dx \right) \\
& \displaystyle = \dfrac{\sqrt{3}}{2\sqrt{2}} \left( \int_{-\frac{1}{2}}^{\frac{1}{2}} \dfrac{1}{\cos\theta_1} \> dx+\int_{-\frac{1}{2}}^{\frac{1}{2}} \dfrac{1}{\cos\theta_2} \> dx \right) \\
& \displaystyle = \dfrac{\sqrt{3}}{2\sqrt{2}} \left( \int_{0}^{\alpha} \dfrac{1}{\cos\theta_1} \dfrac{\sqrt{3}}{2}\dfrac{d\theta_1}{\cos^2\theta_1}+\int_{-\alpha}^{0} \dfrac{1}{\cos\theta_2} \dfrac{\sqrt{3}}{2}\dfrac{d\theta_2}{\cos^2\theta_2} \right) \\
& \displaystyle = \dfrac{3}{4\sqrt{2}} \left( \int_{0}^{\alpha} \dfrac{d\theta_1}{\cos^3\theta_1}+\int_{-\alpha}^{0} \dfrac{d\theta_2}{\cos^3\theta_2} \right) \\
& \displaystyle = \dfrac{3}{4\sqrt{2}} \int_{-\alpha}^{\alpha} \dfrac{d\theta}{\cos^3\theta} \\
& \displaystyle = \dfrac{3}{2\sqrt{2}} \int_{0}^{\alpha} \dfrac{d\theta}{\cos^3\theta} \\
\end{array} \]
ここで,\(\dfrac{1}{\cos^3\theta}\)の不定積分を考える。
\[\begin{array}{rl}
\displaystyle \int \dfrac{d\theta}{\cos^3\theta}
& \displaystyle =\int \dfrac{1}{\cos^2\theta} \dfrac{1}{\cos\theta} d\theta \\
& \displaystyle =\int (\tan\theta)’\dfrac{1}{\cos\theta} d\theta \\
& \displaystyle = \dfrac{\tan\theta}{\cos\theta}-\int \tan\theta \left( \dfrac{1}{\cos\theta} \right)’ d\theta \\
& \displaystyle = \dfrac{\tan\theta}{\cos\theta}-\int \tan\theta \dfrac{\tan\theta}{\cos\theta} d\theta \\
& \displaystyle = \dfrac{\tan\theta}{\cos\theta}-\int \tan^2\theta \dfrac{1}{\cos\theta} d\theta \\
& \displaystyle = \dfrac{\tan\theta}{\cos\theta}-\int \left( \dfrac{1}{\cos^2\theta}-1 \right) \dfrac{1}{\cos\theta} d\theta \\
& \displaystyle = \dfrac{\tan\theta}{\cos\theta}-\int \dfrac{1}{\cos^3\theta} \> d\theta + \int \dfrac{1}{\cos\theta} \> d\theta \\
\end{array}\]
\[\begin{array}{rl}
\displaystyle 2\int \dfrac{1}{\cos^3\theta} \> d\theta
& \displaystyle = \dfrac{\tan\theta}{\cos\theta}+\int \dfrac{1}{\cos\theta} \> d\theta \\
\displaystyle \int \dfrac{1}{\cos^3\theta} \> d\theta
& \displaystyle = \dfrac{1}{2}\dfrac{\tan\theta}{\cos\theta}+\dfrac{1}{2}\int \dfrac{1}{\cos\theta} \> d\theta
\end{array}\]
さらに,\(\dfrac{1}{\cos\theta}\)の不定積分を考えると,
\[\begin{array}{rl}
\displaystyle \int \dfrac{d\theta}{\cos\theta}
& \displaystyle = \int \dfrac{\cos\theta}{\cos^2\theta} \> d\theta \\
& \displaystyle = \int \dfrac{\cos\theta}{1-\sin^2\theta} \> d\theta \\
& \displaystyle = \int \dfrac{\cos\theta}{(1+\sin\theta)(1-\sin\theta)} \> d\theta \\
& \displaystyle = \int \dfrac{1}{2} \left( \dfrac{\cos\theta}{1+\sin\theta} + \dfrac{\cos\theta}{1-\sin\theta}\right) \> d\theta \\
& \displaystyle = \dfrac{1}{2} \int \left( \dfrac{(1+\sin\theta)’}{1+\sin\theta}-\dfrac{(1-\sin\theta)’}{1-\sin\theta} \right) \> d\theta \\
& \displaystyle = \dfrac{1}{2} \left( \log|1+\sin\theta|-\log|1-\sin\theta| \right)+C \\
& \displaystyle = \dfrac{1}{2} \log\dfrac{1+\sin\theta}{1-\sin\theta}+C
\end{array}\]
なので,
\[\begin{array}{rl}
\displaystyle \int \dfrac{1}{\cos^3\theta} \> d\theta
& \displaystyle = \dfrac{1}{2}\dfrac{\tan\theta}{\cos\theta}+\dfrac{1}{4}\log\dfrac{1+\sin\theta}{1-\sin\theta}+C
\end{array}\]
\(\tan\alpha=\dfrac{2}{\sqrt{3}}\)より\(\sin\alpha=\dfrac{2}{\sqrt{7}}\),\(\cos\alpha=\dfrac{\sqrt{3}}{\sqrt{7}}\)なので,
\[\begin{array}{rl}
I & \displaystyle = \dfrac{3}{2\sqrt{2}} \int_{0}^{\alpha} \dfrac{d\theta}{\cos^3\theta} \\
& =\dfrac{3}{2\sqrt{2}} \left[ \dfrac{1}{2}\dfrac{\tan\theta}{\cos\theta}+\dfrac{1}{4}\log\dfrac{1+\sin\theta}{1-\sin\theta} \right]_0^\alpha \\
& =\dfrac{3}{2\sqrt{2}} \left\{ \left( \dfrac{1}{2}\dfrac{2}{\sqrt{3}}\dfrac{\sqrt{7}}{\sqrt{3}}+\dfrac{1}{4}\log\dfrac{1+\frac{2}{\sqrt{7}}}{1-\frac{2}{\sqrt{7}}} \right)-0 \right\} \\
& =\dfrac{3}{2\sqrt{2}} \left( \dfrac{\sqrt{7}}{3}+\dfrac{1}{4}\log\dfrac{\sqrt{7}+2}{\sqrt{7}-2} \right) \\
& =\dfrac{\sqrt{14}}{4}+\dfrac{3\sqrt{2}}{16}\log\dfrac{\sqrt{7}+2}{\sqrt{7}-2}
\end{array}\]
問題は次の動画から。自分でも解いてみたので解答を残すことにしました。
\( \displaystyle \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{\left( x+\dfrac{1}{2} \right)^2+\dfrac{3}{4}} dx\)からの変数変換で,\(y=x+\dfrac{1}{2}\)とおいて,\(y+\sqrt{y^2+\dfrac{3}{4}} = t\)とするのは初めて見た気がするので,覚えておこうと思います。
\[\begin{array}{rl}
y+\sqrt{y^2+\dfrac{3}{4}} & =t \\
\sqrt{y^2+\dfrac{3}{4}} & =t-y \\
y^2+\dfrac{3}{4} & =t^2-2ty+y^2 \\
2ty & =t^2-\dfrac{3}{4} \\
8ty & =4t^2-3 \\
y & =\dfrac{4t^2-3}{8t} \\
\dfrac{dy}{dt} & = \dfrac{8t \cdot 8t-(4t^2-3) \cdot 8}{64t^2} \\
&= \dfrac{8t^2-4t^2+3}{8t^2} \\
&= \dfrac{4t^2+3}{8t^2} \\
\end{array}\]
となるから,
\[\begin{array}{rl}
\displaystyle \int_{-\frac{1}{2}}^{\frac{1}{2}} \sqrt{\left( x+\dfrac{1}{2} \right)^2+\dfrac{3}{4}} dx
& \displaystyle = \int_0^1 \sqrt{y^2+\dfrac{3}{4}} \> dy \\
& \displaystyle = \int_{0}^{1} (t-y) dy \\
& \displaystyle = \int_{\frac{\sqrt{3}}{2}}^{1+\frac{\sqrt{7}}{2}} \left( t-\dfrac{4t^2-3}{8t} \right) \dfrac{4t^2+3}{8t^2} dt \\
& \displaystyle = \int_{\frac{\sqrt{3}}{2}}^{1+\frac{\sqrt{7}}{2}} \dfrac{4t^2+3}{8t} \dfrac{4t^2+3}{8t^2} dt \\
& \displaystyle = \int_{\frac{\sqrt{3}}{2}}^{1+\frac{\sqrt{7}}{2}} \dfrac{(4t^2+3)^2}{64t^3} dt \\
& \displaystyle = \int_{\frac{\sqrt{3}}{2}}^{1+\frac{\sqrt{7}}{2}} \dfrac{16t^4+24t^2+9}{64t^3} dt \\
& \displaystyle = \dfrac{1}{64} \int_{\frac{\sqrt{3}}{2}}^{1+\frac{\sqrt{7}}{2}} (16t+24t^{-1}+9t^{-3}) dt \\
& \displaystyle = \dfrac{1}{64} \left[ 8t^2+24\log|t|-\dfrac{9}{2}t^{-2} \right]_{\frac{\sqrt{3}}{2}}^{1+\frac{\sqrt{7}}{2}} \\
& = \cdots \\
& = \dfrac{\sqrt{7}}{4}+\dfrac{3}{8}\log\dfrac{2+\sqrt{7}}{\sqrt{3}}
\end{array}\]
式は複雑ですが,三角関数を経由しないのがいいですね。
相関係数から散布図を作りたい
任意の相関係数\(r\)から,それらしい散布図を作ろうと考えました。

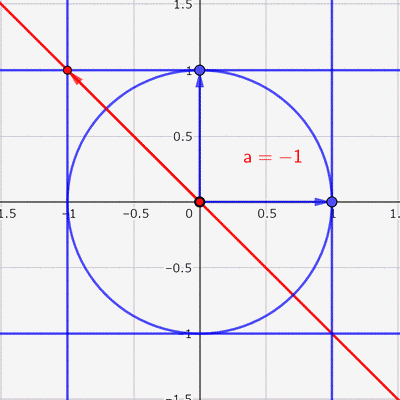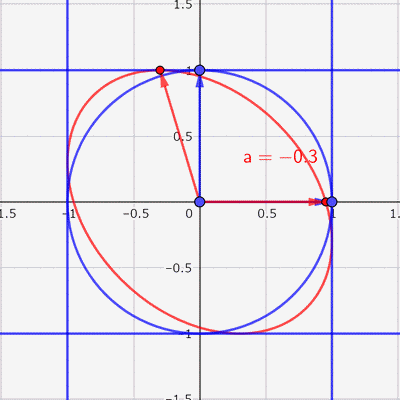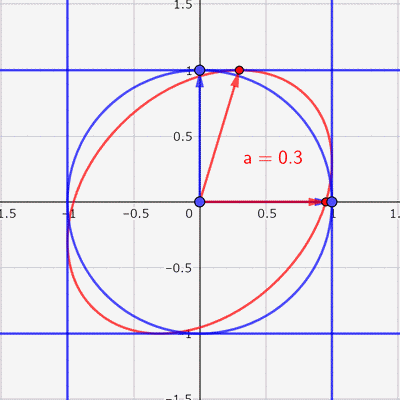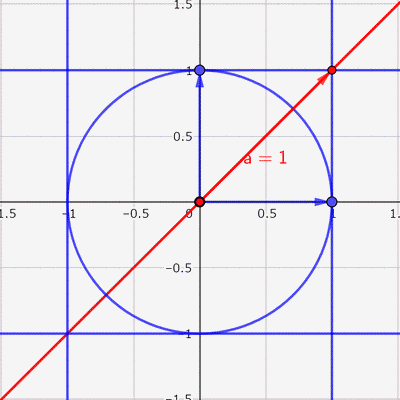
思いついたのが,半径\(1\)の円を基底変換して,いい感じの形を作ればいいのではないかと。
\(-1 \leqq x \leqq 1\),\(-1 \leqq y \leqq 1\)の範囲に収めるためには,
\[ \left[ \begin{array}{cc} \sqrt{1 – a^2} & a \\ 0 & 1 \end{array} \right] \]
の\(1\)次変換をすれば良さそうです。
この式は次のように考えました。相関係数が大きくなると上向きのベクトルが右上の方に変化すると考えて,右方向に動く量を\(a\)とすれば,
\[ \left[ \begin{array}{cc} b & a \\ 0 & 1 \end{array} \right] \left[ \begin{array}{c} \cos \theta \\ \sin \theta \end{array} \right] = \left[ \begin{array}{c} b \cos \theta + a \sin \theta \\ \sin \theta \end{array} \right] \]
の変換を考えて,\(y\)座標は必ず\(-1 \leqq y \leqq 1\)になるから,問題は\(x\)座標で,
\[b \cos \theta + a \sin \theta = \sqrt{a^2 + b^2} \left( \cos \theta \cdot \dfrac{b}{\sqrt{a^2 + b^2}} + \sin \theta \cdot \dfrac{a}{\sqrt{a^2 + b^2}} \right) \]
となるから,
\[ \cos \alpha = \dfrac{b}{\sqrt{a^2 + b^2}}, \sin \alpha = \dfrac{a}{\sqrt{a^2 + b^2}} \]
と置けば,
\[ b \cos \theta + a \sin \theta = \sqrt{a^2 + b^2} \cos(\theta-\alpha) \]
なので,
\[ -\sqrt{a^2 + b^2} \leqq b \cos \theta + a \sin \theta \leqq \sqrt{a^2 + b^2} \]
これより,\(\sqrt{a^2 + b^2} = 1 \)であるから,\(a^2 + b^2 = 1\)なので,
\[ b = \sqrt{1-a^2}\]
これより,媒介変数表示で表すと,次のようになります。\(t\)の範囲は\(0 \leqq t \leqq 2\pi \)
\[ \left\{ \begin{array}{l} x = \sqrt{1-a^2}\cos t + a\sin t \\ y = \sin t \end{array} \right. \]
あとは,この赤い楕円の中に点をランダムに打てば,いい感じの散布図ができそうです。
相関係数が\(a\)と同じだったらうれしいなと思って,計算してみました。
この赤い楕円に囲まれた部分にランダムに点を打つことを考えます。横軸をX,縦軸をYとして,Xの分散,Yの分散,XとYの共分散を求めていきます。まず,赤い楕円に囲まれた部分の面積は,青い円を横に\(\sqrt{1-a^2}\)倍しただけなので,
\[\pi\sqrt{1-a^2}\]
です。また,さきほどの媒介変数表示の式を陽関数に変換してみると,
\[ \begin{array}{l} y(x) = ax \pm \sqrt{1-a^2}\sqrt{1-x^2} \\ x(y) = ay \pm \sqrt{1-a^2}\sqrt{1-y^2} \end{array} \]
と表すことができるので,
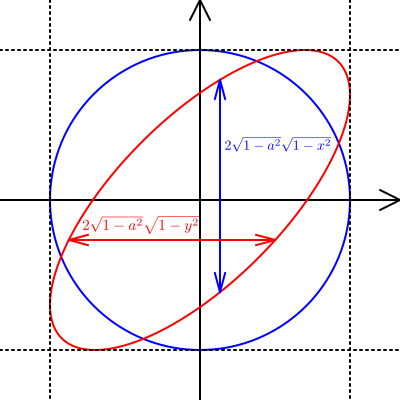
\(x\)方向と\(y\)方向でそれぞれの幅\(w_x(y)\),\(w_y(x)\)を調べると,
\[ \begin{array}{l} w_x(y) = 2\sqrt{1-a^2}\sqrt{1-y^2} \\ w_y(x) = 2\sqrt{1-a^2}\sqrt{1-x^2} \end{array} \]
ですから,XとYの確率密度関数\(f_x(x)\),\(f_y(y)\)は,これらの値を面積で割ることで,
\[ \begin{array}{l} f_x(x) = \dfrac{2\sqrt{1-a^2}\sqrt{1-x^2}}{\pi\sqrt{1-a^2}} = \dfrac{2}{\pi}\sqrt{1-x^2} \\ f_y(y) = \dfrac{2\sqrt{1-a^2}\sqrt{1-y^2}}{\pi\sqrt{1-a^2}} = \dfrac{2}{\pi}\sqrt{1-y^2} \end{array} \]
のように求めることができます。つまり,どちらも\(g(t) = \dfrac{2}{\pi}\sqrt{1-t^2}\)と表せます。
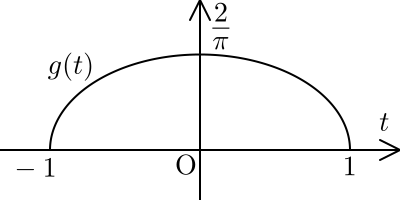
よって,分散は,
\[ \begin{array}{rl}
E[X] & = E[Y] = \displaystyle \int_{-1}^1 xg(x) dx = 0 \\
V[X] & = V[Y] = E[(X-0)^2]=E[X^2] = \displaystyle \int_{-1}^1 x^2g(x) dx \\
& = \displaystyle \dfrac{2}{\pi} \int_{-1}^1 x^2\sqrt{1-x^2} dx \\
& = \displaystyle \dfrac{2}{\pi} \int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \sin^2\theta \cos \theta \cdot \cos \theta d\theta \\
& = \displaystyle \dfrac{2}{\pi} \int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \dfrac{1}{4} \sin^2 2\theta d\theta \\
& = \displaystyle \dfrac{1}{2\pi} \int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \dfrac{1-\cos 4\theta}{2} d\theta \\
& = \displaystyle \dfrac{1}{4\pi} \left[ \theta-\dfrac{1}{4}\sin 4\theta \right]_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \\
& = \dfrac{1}{4\pi} \left\{ \dfrac{\pi}{2} – \left( \dfrac{\pi}{2} \right) \right\} = \dfrac{1}{4}
\end{array}\]
のように計算できます。また,同時確率密度関数\( f_{xy}(x,y) \)は,
\( -1 \leqq x \leqq 1, ax-\sqrt{1-a^2}\sqrt{1-x^2} \leqq y \leqq ax+\sqrt{1-a^2}\sqrt{1-x^2} \)の範囲で\(\dfrac{1}{\pi\sqrt{1-a^2}}\),
それ以外で\(0\)をとる関数なので,共分散は
\[ \begin{array}{rl}
Cov(X,Y) & = E[(X-E[X])(Y-E[Y])] = E[XY] \\
& = \displaystyle \int_{-1}^1 \int_{ax-\sqrt{1-a^2}\sqrt{1-x^2}}^{ax+\sqrt{1-a^2}\sqrt{1-x^2}} xy \cdot \dfrac{1}{\pi\sqrt{1-a^2}} dy dx \\
& = \displaystyle \dfrac{1}{\pi\sqrt{1-a^2}} \int_{-1}^1 x \left[ \dfrac{1}{2}y^2 \right]_{ax-\sqrt{1-a^2}\sqrt{1-x^2}}^{ax+\sqrt{1-a^2}\sqrt{1-x^2}} dx \\
& = \displaystyle \dfrac{1}{\pi\sqrt{1-a^2}} \int_{-1}^1 x \cdot 2ax\sqrt{1-a^2}\sqrt{1-x^2} dx \\
& = \displaystyle \dfrac{2a}{\pi} a \int_{-1}^1 x^2\sqrt{1-x^2} dx \\
& = \dfrac{2a}{\pi} \cdot \dfrac{\pi}{8} = \dfrac{a}{4}
\end{array} \]
したがって,相関係数\(r\)は,
\[r = \dfrac{Cov(X,Y)}{\sqrt{V[X] \cdot V[Y]}} = \dfrac{\frac{a}{4}}{\sqrt{\frac{a}{4} \cdot \frac{a}{4}}} = a\]
となります。
実際に作ってみると,\(r=0.7\)の場合は,

こんな感じになりました。ただ,点の数が少ないので,相関係数の値が結構ぶれてしまいます。試行回数を増やせば,目的の散布図は作成できそうです。
2nCn/2が奇数となるnを求めよ
問題 \(\dfrac{{}_{2n}{\rm C}_n}{2}\)が奇数となる\(n\)をすべて求めよ。
\({}_{2n}{\rm C}_n = \dfrac{(2n)!}{n!n!}\)と表せるので,\(\dfrac{{}_{2n}{\rm C}_n}{2}\)が奇数となるには,\((2n)!\)に含まれる素因数\(2\)の数から\((n!)^2\)に含まれる素因数\(2\)の数を引いて\(1\)となればよい。
つまり,\(v(m)\)を\(m\)に含まれる素因数\(2\)の数を表す関数とすると,$$v((2n)!) – 2v(n!) = 1$$が成り立てばよい。
ここで,\(v(m!)\)は,$$\begin{array}{r@{\>}l} v(m!) & = \left\lfloor \dfrac{m}{2} \right\rfloor + \left\lfloor \dfrac{m}{4} \right\rfloor + \left\lfloor \dfrac{m}{2^3} \right\rfloor + \cdots + \left\lfloor \dfrac{m}{2^i} \right\rfloor + \cdots \\ & = \displaystyle \sum_{i = 1}^\infty \left\lfloor \dfrac{m}{2^i} \right\rfloor \end{array}$$と計算できる。
整数\(m_1, m_2, \cdots, m_k\)\((m_1 > m_2 > \cdots >m_k)\)を用いて,
\( n = 2^{m_1} + 2^{m_2} + \cdots + 2^{m_k} \)とすると,$$v(n!) = \displaystyle \sum_{i=1}^\infty \left\lfloor \dfrac{2^{m_1} + 2^{m_2} + \cdots + 2^{m_k}}{2^i} \right\rfloor $$と表せる。
ここで,\(m_1>m_2>\cdots>m_s \geq i > m_t > \cdots > m_k \)となるとき,
\(2^{m_t}+2^{m_{t+1}}+\cdots+2^{k-1}+2^k<2^i\)なので,\(\dfrac{2^{m_t}+2^{m_{t+1}}+\cdots+2^{k}}{2^i} < 1\)であるから,$$ \begin{array}{r@{\>}l} \left\lfloor \dfrac{2^{m_1}+2^{m_2}+\cdots+2^{m_k}}{2^i} \right\rfloor & = 2^{m_1-i}+2^{m_2-i}+\cdots+2^{m_s-i} \\ & = \left\lfloor \dfrac{2^{m_1}}{2^i} \right\rfloor + \left\lfloor \dfrac{2^{m_2}}{2^i} \right\rfloor + \cdots + \left\lfloor \dfrac{2^{m_k}}{2^i} \right\rfloor \end{array} $$と表せるから,
$$\begin{array}{rl} v(n!) & = \displaystyle \sum_{i=1}^\infty \left( \left\lfloor \dfrac{2^{m_1}}{2^i} \right\rfloor + \left\lfloor \dfrac{2^{m_2}}{2^i} \right\rfloor + \cdots + \left\lfloor \dfrac{2^{m_k}}{2^i} \right\rfloor \right) \\ & = \displaystyle \sum_{i=1}^\infty \left\lfloor \dfrac{2^{m_1}}{2^i} \right\rfloor + \sum_{i=1}^\infty \left\lfloor \dfrac{2^{m_2}}{2^i} \right\rfloor + \cdots + \sum_{i=1}^\infty \left\lfloor \dfrac{2^{m_k}}{2^i} \right\rfloor \\ & = (2^{m_1-1}+2^{m_1-2}+\cdots+2^0) + (2^{m_2-1}+2^{m_2-2} +\cdots+2^0) + \cdots \\ & = \dfrac{2^{m_1}-1}{2-1} + \dfrac{2^{m_2}-1}{2 – 1} + \cdots + \dfrac{2^{m_k}-1}{2 – 1} \\ & = 2^{m_1} + 2^{m_2} + \cdots + 2^{m_k} – k \end{array}$$
\(v((2n)!)\)についても,同様に考えると,$$v((2n)!) = 2^{m_1+1} + 2^{m_2+1} + \cdots +2^{m_k+1}-k$$と表せる。
よって,$$\begin{array}{l} v((2n)!) – 2v(n!) \\ = (2^{m_1+1}+\cdots+2^{m_k+1}-k)-2(2^{m_1}+\cdots+2^{m_k+1}-k) \\ = (2^{m_1+1}+\cdots+2^{m_k+1}-k)-(2^{m_1+1}+\cdots+2^{m_k+1}-2k) \\ =k \end{array}$$となる。
したがって,条件を満たすには\(k = 1\)でなければならないから,\(0\)以上の整数\(j\)を使って,\(n=2^j\)と表せるときだけ\(\dfrac{{}_{2n}{\rm C}_n}{2}\)が奇数になる。
この問題は下の動画を見て,ChatGPTで解けるかなと思ってやってみました。最初は\(n=1\)のときのみを答えとしていてうまく答えを出すことはできませんでした。模範解答は\(n=2^j\)だよと教えると,間違っていましたが,それらしい解答を提示するようになりました。上の解答はChatGPTが示した解答を大幅に加筆,修正したものです。
\({}_{2n}{\rm C}_n\)の素因数\(2\)の数が\(n\)を\(2\)進数表記をしたときの\(1\)の数と等しいのは,面白い性質です。ChatGPT(3.5)で数学はできませんが,ChatGPT(4)であれば簡単な問題を解いてくるようになりました。提示してくる解答は正しいとは限りませんが,よいアイデアを出すこともあるので面白いですね。
NASを導入してみた
これまで,Windowsサーバーでファイル交換をしていましたが,容量不足なので,NASを導入してみようと思いました。初めてのNAS導入ということで,安価なSynology製のDISKSTATION DS220です。
ストレージはWesternDigital製の8TBのHDD2台でRAID1にします。

HDDが同時に故障するのを防ぐためにロットをずらすことがよくやられているということで、ドスパラとツクモでそれぞれ購入。左は2023.06.05,右は2023.3.31なのでロット違いなものが入手できました。

導入はNASのふたを外して,HDDをさして,横からねじ止めをして終了。
自作パソコンを作ったことがある人は楽に作れます。

難なく完成。ソフトもしっかり作られているので,ネットワークの設定も簡単にできました。

ブログ開設
今後はこちらに日々の出来事を書き込みたいと思います。